Buying on Margin
Investors can trade through a broker in one of two ways: they can either
use their own money, or they can open a margin account with the broker.
In this case, they use the broker's money, which he lends to them at a
stipulated rate of interest. The advantage of borrowing the money in this
manner from the broker is that the broker can lend to the trader at a lower
rate of interest, since he has the securities in his possession and can
use them as collateral.
Since the securities purchased by the trader function as collateral
for the loan, the current market value of the loan is crucial vis-à-vis
the amount lent. In order that the market value of the securities does
not fall too low, the broker requires that the ratio of market value to
the loan always exceed a certain proportion. Alternatively, the customer's
equity in the securities as a proportion of the total value of the securities
cannot fall below a specified proportion. This proportion is termed the
margin requirement. Currently, the legal margin requirement on all stocks
is 50%; i.e. at least 50% of the price of the securities should be financed
by the customer himself. However, the broker can demand a higher margin
level. This margin requirement at the time of initial purchase, which is
termed the initial margin is larger than the margin requirement
at a later time.
The initial margin requirement is given by the ratio:
 . .
The actual margin at any time is given by:
 . The minimum value of the
AM that is permitted by the broker is called the maintenance margin
(MM). Here's an example of how the maintenance margin comes into play. . The minimum value of the
AM that is permitted by the broker is called the maintenance margin
(MM). Here's an example of how the maintenance margin comes into play.
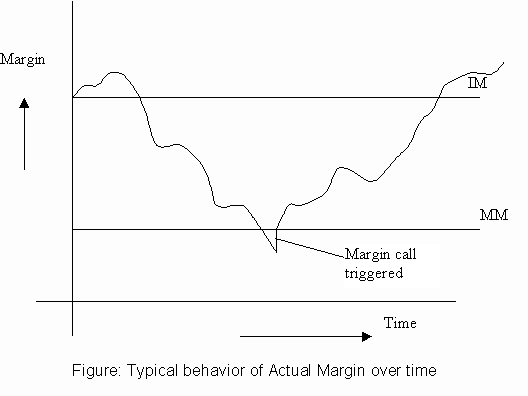
Suppose, to begin with, the customer borrows 50% of the value of the
stocks purchased. As the market value of the stocks drops, the actual margin
will drop until, if the price drops enough, it will drop below the MM.
As soon as this happens, the account is said to be undermargined.
At this point, a margin call will be made by the broker, requiring the
investor to add more liquid funds to his account, until the AM is greater
than or equal to the MM. The normal practice among brokers is only to require
enough additional funds for the AM to come up to the MM level. However,
it is possible for the broker to require that, once the AM has dropped
below the MM, it should be brought up to some higher margin level, such
as the IM level.
On the other hand, if the market value of the assets rises, the account
becomes overmargined. If the actual margin is greater than the IM, it is
unrestricted, and the trader can withdraw the excess of the funds above
the IM. If the AM is between the IM and the MM, no margin calls are made,
but no funds can be withdrawn either. However, different brokerage houses
have different rules, and some of them may permit cash withdrawals provided
maintenance margin limits are met. Conversely, other brokerage houses
do not actually permit excess funds to be withdrawn in cash, but will allow
them to be used for other transactions. Finally, some brokerage houses
will set aside any funds that exceed initial margin requirements, and will
allow them to be withdrawn, even if subsequently actual margin drops below
the initial margin level.
Example 1:
Consider the following example: Martin buys 200 shares of MBIA stock at
32 3/4, on margin. The initial margin requirement is 50%. Hence he needs
to put in 0.5(32.75 x 200) = $3275 of his own money. At this point, the
AM = IM. If the stock price should drop far enough, Martin would receive
a margin call. How low can the price drop without triggering a margin call?
Suppose the maintenance margin requirement is 35%. Then, the price could
drop up to the value of P that satisfies the equation:
(200P - 3275)/(200P) = 0.35.
where the assets in the account equal 200P, and the amount of the loan
is $3275. Solving, we get a value of P = 25.19. Now suppose the price drops
to $22. What is the amount of the additional margin that is necessary for
the AM to get back up to the MM level? This can be computed by solving
the following equation:
(200 x 22 + Y -3275)/(200 x 22) = 0.35
Solving, we get $415. At this point, the actual margin would be equal
to (4400+415-3275)/(4400) = 0.35 as required.
Example 2:
Q. Bret Barakett has a margin account and deposits $50,000. Assuming
the prevailing initial margin requirement is 40%, commissions are ignored,
and Reebok is selling at $35 per share, how many shares can Brett purchase
using the maximum allowable margin?
Solution: Brett can buy 50000/(.4)(35) or 3571.43 shares
Q. What is Brett's profit/loss if the price of Reebok stock falls to
$25?
Solution: If the price falls to $25, the loss is (35-25)(3571.43)
or 35714.30
Q. If the maintenance margin is 30%, to what price can Reebok fall before
Brett will receive a margin call?
Solution: The price can fall up P, where P is given by
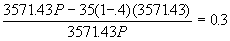 , or P = $30. , or P = $30.
Q. How much additional money must Brett deposit if the stock price drops
to $5 below the level computed in c) above, and he is required to bring
up the actual margin on his account to the maintenance margin?
Solution: If the price falls to $25, he needs to have 0.3(25)(3571.43)
or $26785.73; he originally had $50,000, of which he lost 35714.30. Hence
he now needs an additional 26785.73 - (50000-35714.30) = $12500.
Alternatively, the broker’s loan is now restricted to 70% of the value
of the securities or .7(25)(3571.43) = 62500. The original loan amount
was 75000. Hence the amount that the customer must pay up is 75000 - 62500
= $12500.
Account Information:
At the beginning:
|
Assets
|
Liabilities
|
| Cash |
$50000.00 |
Equity |
$50000.00 |
| Total |
$50000.00 |
Total |
$50000.00 |
Margin = 50000/50000 = 100%
After the shares are purchased:
|
Assets
|
Liabilities
|
| 3571.43 shares of Reebok at $35 each. |
125000.00 |
Loan |
75000.00 |
| |
|
Equity |
50000.00 |
| Total |
125000.00 |
Total |
125000.00 |
Margin = 50000/125000 = 40%
After the share price falls to $25:
|
Assets
|
Liabilities
|
| 3571.43 shares of Reebok at $25 each. |
89285.71 |
Loan |
75000.00 |
| |
|
Equity |
14285.71 |
| Total |
89285.71 |
Total |
89285.71 |
Margin = 14285.71/89285.71 = 16%
After the margin call is paid:
|
Assets
|
Liabilities
|
| 3571.43 shares of Reebok at $25 each. |
89285.71 |
Loan (75000.00 - 12500) |
62500.00 |
| |
|
Equity |
26785.71 |
| Total |
89285.71 |
Total |
89285.71 |
Margin = 26785.71/89285.71 = 30%
Short Sales
The Logistics of a Short Sale
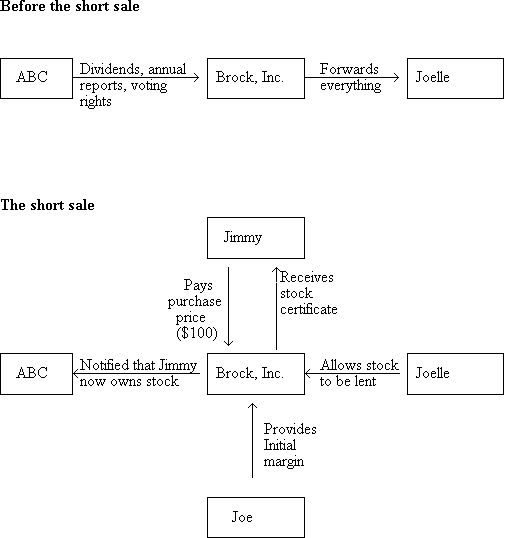
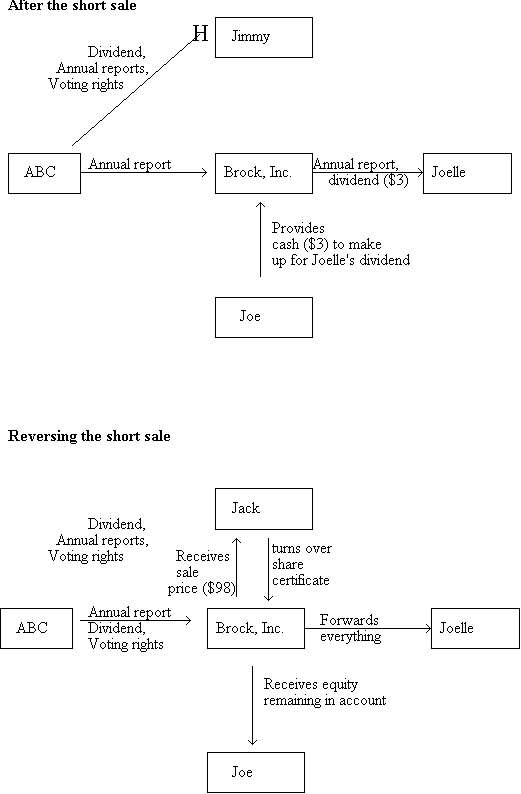
Margin Computation with short sales:
Since a short seller of a stock has the corresponding obligation of buying
back the stock at some point in the market, and returning it to the broker,
the broker does not typically permit the shortseller to withdraw the proceeds
of the short sale. In fact, he requires the shortseller to deposit a further
sum of money in case the stock price rises, and the short seller should
need additional funds to buy back the stock.
In the case of a short sale, the trader is borrowing, not cash, but
rather securities, and margin rules apply here as well. The margin quantities,
in this case is computed as follows:
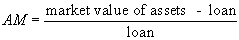 , where the
loan amount is the market value of the security borrowed at the time that
the margin is being computed. Hence, if Tina sold short 200 shares of WMT
(Wal-Mart Stores, Inc.), when the stock was selling at $25, assuming a
50% initial margin, she would have had to put $25 x 200 x 50% = $2500 into
her account., so that the loan of 200 x $25 or $5000 satisfies , where the
loan amount is the market value of the security borrowed at the time that
the margin is being computed. Hence, if Tina sold short 200 shares of WMT
(Wal-Mart Stores, Inc.), when the stock was selling at $25, assuming a
50% initial margin, she would have had to put $25 x 200 x 50% = $2500 into
her account., so that the loan of 200 x $25 or $5000 satisfies 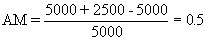 , where the first term of $5000 in the numerator refers to the original
proceeds of the short sale of stock, the second term of $2500 refers to
the money that was put up as margin, and the third term of $5000 refers
to the value of the loan made to the trader. Just as in the case of a margin
purchase, we have maintenance margin requirements here, as well. Suppose
this is 25%. Then, if the market value of the stock rises to $35, the actual
margin, now, is , where the first term of $5000 in the numerator refers to the original
proceeds of the short sale of stock, the second term of $2500 refers to
the money that was put up as margin, and the third term of $5000 refers
to the value of the loan made to the trader. Just as in the case of a margin
purchase, we have maintenance margin requirements here, as well. Suppose
this is 25%. Then, if the market value of the stock rises to $35, the actual
margin, now, is 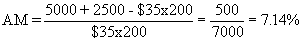 . Since this is less than 25%, Tina must put up enough money to bring up
the margin to the required initial margin of 50%, once again. This amount
of money can be computed by solving the equation . Since this is less than 25%, Tina must put up enough money to bring up
the margin to the required initial margin of 50%, once again. This amount
of money can be computed by solving the equation 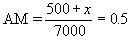 , which works out to $3000. , which works out to $3000.
Normally, the broker will also allow the trader to use Treasury bills
to meet margin calls since they are very liquid and essentially equivalent
to cash. However, this depends on the agreement between the trader and
the broker.
If the customer has purchased a stock on margin and short sold another
stock, the total dollar margin required on the account is computed by aggregating
the dollar amounts required for each separately. Thus, if we require a
maintenance margin of 25% for both short sales and margin purchases, this
means the following. For the margin purchase, we require  , i.e. equity must be greater than or equal to 0.25(current market
value of assets). For the short sale, we require , i.e. equity must be greater than or equal to 0.25(current market
value of assets). For the short sale, we require 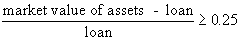 , i.e. equity must be at least 25% of the loan amount. Hence if the current
market value of securities purchased on margin is $200 with $165 borrowed
from the broker, and if the current market value of securities shortsold
is $160, the total amount of equity that must be held in the customer's
account is 0.25(200) + 0.25(160) = $90. If the shortsold securities
were originally trading at $130 at the time they were shortsold, the amount
of cash realized from the short sale would have been 1.5(130) = $195, assuming
that initial margin was 50%; since the current market value of the shortsold
securities is $160, the amount of equity is 195 - 160 = $35 on the short
sale side. On the margin purchase side, the amount of equity is 200
- 165 = 35, for a total equity of 35 + 35 = 70. Hence, the difference
of $20 (90 - 70) must be deposited in cash. , i.e. equity must be at least 25% of the loan amount. Hence if the current
market value of securities purchased on margin is $200 with $165 borrowed
from the broker, and if the current market value of securities shortsold
is $160, the total amount of equity that must be held in the customer's
account is 0.25(200) + 0.25(160) = $90. If the shortsold securities
were originally trading at $130 at the time they were shortsold, the amount
of cash realized from the short sale would have been 1.5(130) = $195, assuming
that initial margin was 50%; since the current market value of the shortsold
securities is $160, the amount of equity is 195 - 160 = $35 on the short
sale side. On the margin purchase side, the amount of equity is 200
- 165 = 35, for a total equity of 35 + 35 = 70. Hence, the difference
of $20 (90 - 70) must be deposited in cash.
Example:
Jaeger Corporation stock currently sells for $60 per share. The initial
margin requirement is 50% and the maintenance margin is 30%. If Willie
Danziger sells short 300 shares of Jaeger stock, to what price can the
stock rise before Willie recieves a margin call?
Solution:
The formula for the actual margin is Percentage margin = Equity/Value
of stock owed.
For our data, we get the equation 0.30 = (27000 - 300P)/300P, since
at the beginning, Willie must have put up 300 x 60 x 0.5 in cash plus 300
x 60 from the original sale of the stock = $27000. Solving our equation,
we get P = $69.23.
After the shares are short-sold:
|
Assets
|
Liabilities
|
| Cash from the sale of 300 shares at $60 each = 18000 plus
9000 (300 x 60 x 0.5) put up additionally in cash |
27000.00 |
Loan (equivalent to the current value of the shares borrowed
on Danziger’s behalf, i.e. 300 x 60) |
18000.00 |
| |
|
Equity |
9000.00 |
| Total |
27000.00 |
Total |
27000.00 |
Margin = 9000/18000 = 50%
After the share price rises to $69.23:
|
Assets
|
Liabilities
|
| Cash |
27000.00 |
Loan (69.23 x 300) |
20769.00 |
| |
|
Equity |
6231.00 |
| Total |
27000.00 |
Total |
27000.00 |
Margin = 6231/20769 = 30%
Go to P.V. Viswanath's Home Page
|